计算几何
大约 1 分钟约 369 字
计算几何
点线关系
点的结构体如下:
struct Point {
double x, y;
};
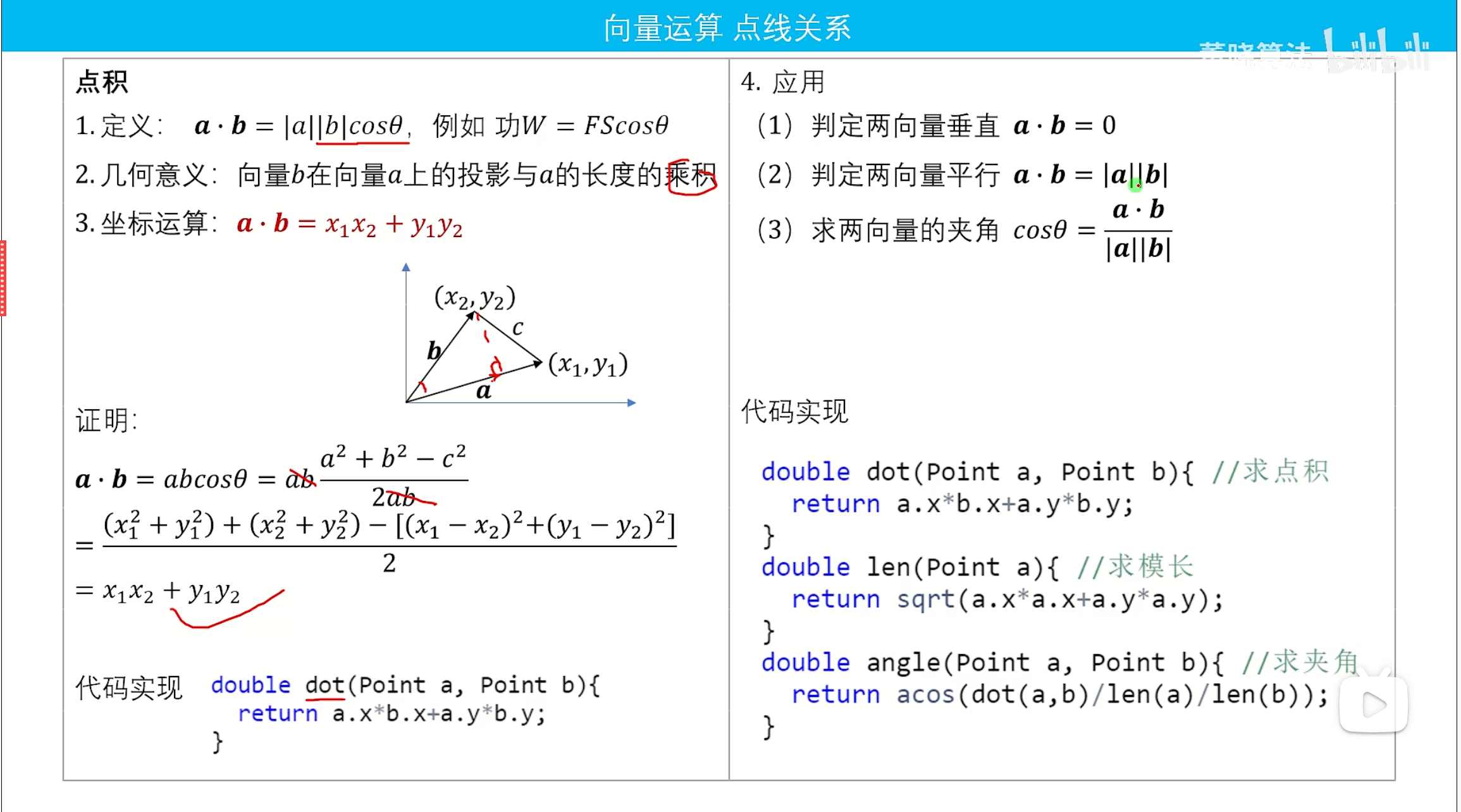
点积
定义:
几何意义:向量b在向量a上的投影与向量a的长度的乘积
坐标运算:
应用
- 两向量垂直:
- 两向量平行:
- 两向量夹角:
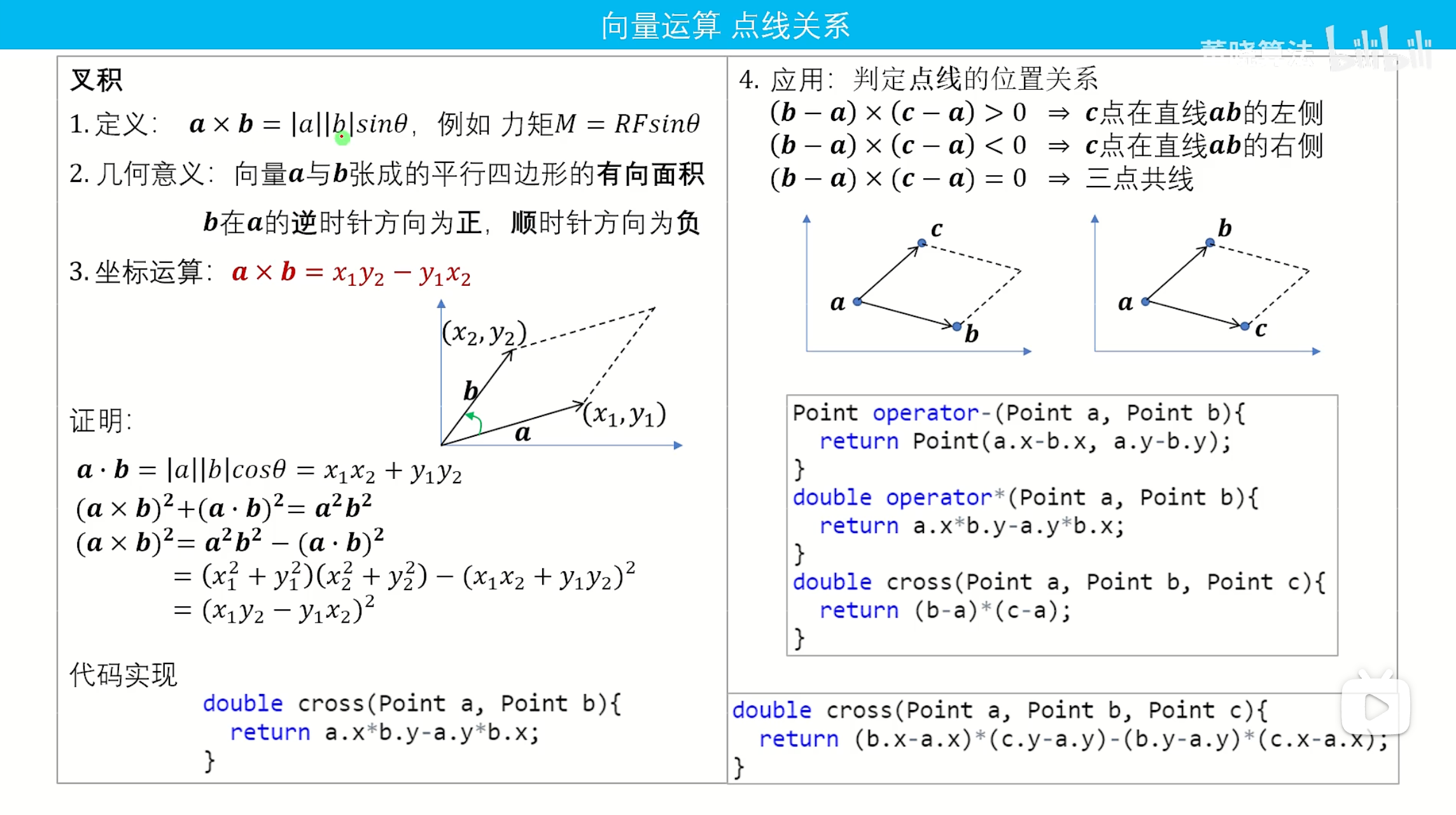
叉积
定义:
几何意义:两个向量形成的平行四边形的面积,b在a逆时针方向为正
运算:
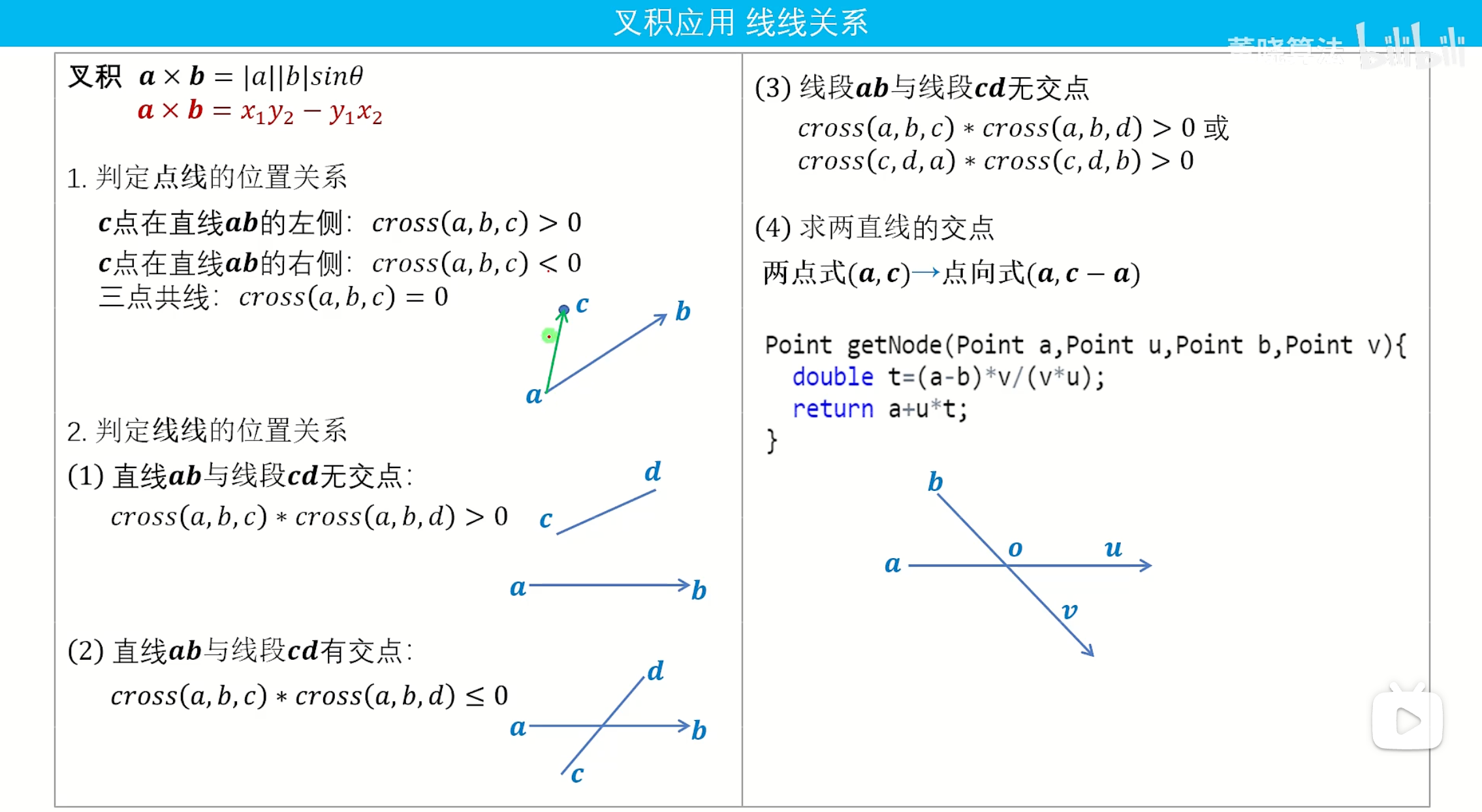
应用:判断点线位置关系:
代码模版
struct Point {
double x, y;
Point operator+(Point b) const {
return {x + b.x, y + b.y};
}
Point operator-(Point b) const {
return {x - b.x, y - b.y};
}
double operator*(Point b) const { //点积
return x * b.x + y * b.y;
}
Point operator*(int t) const {
return {x * t, y * t};
}
double operator&(Point b) const {//叉积
return x * b.y - y * b.x;
}
};
double len(Point a) { //模
return sqrt(a.x * a.x + a.y * a.y);
}
double angle(Point a, Point b) { //cos夹角
double t = a * b / len(a) / len(b);
return acos(t);
}
double cross(Point a, Point b, Point c) { //两个向量叉积
return (c - a) & (b - a);//>0 c在ab左侧 =0 共线
}
double dis(Point a, Point b) {
double t = (a.x - b.x) * (a.x - b.x) + (a.y - b.y) * (a.y - b.y);
return sqrt(t);
}
线线关系
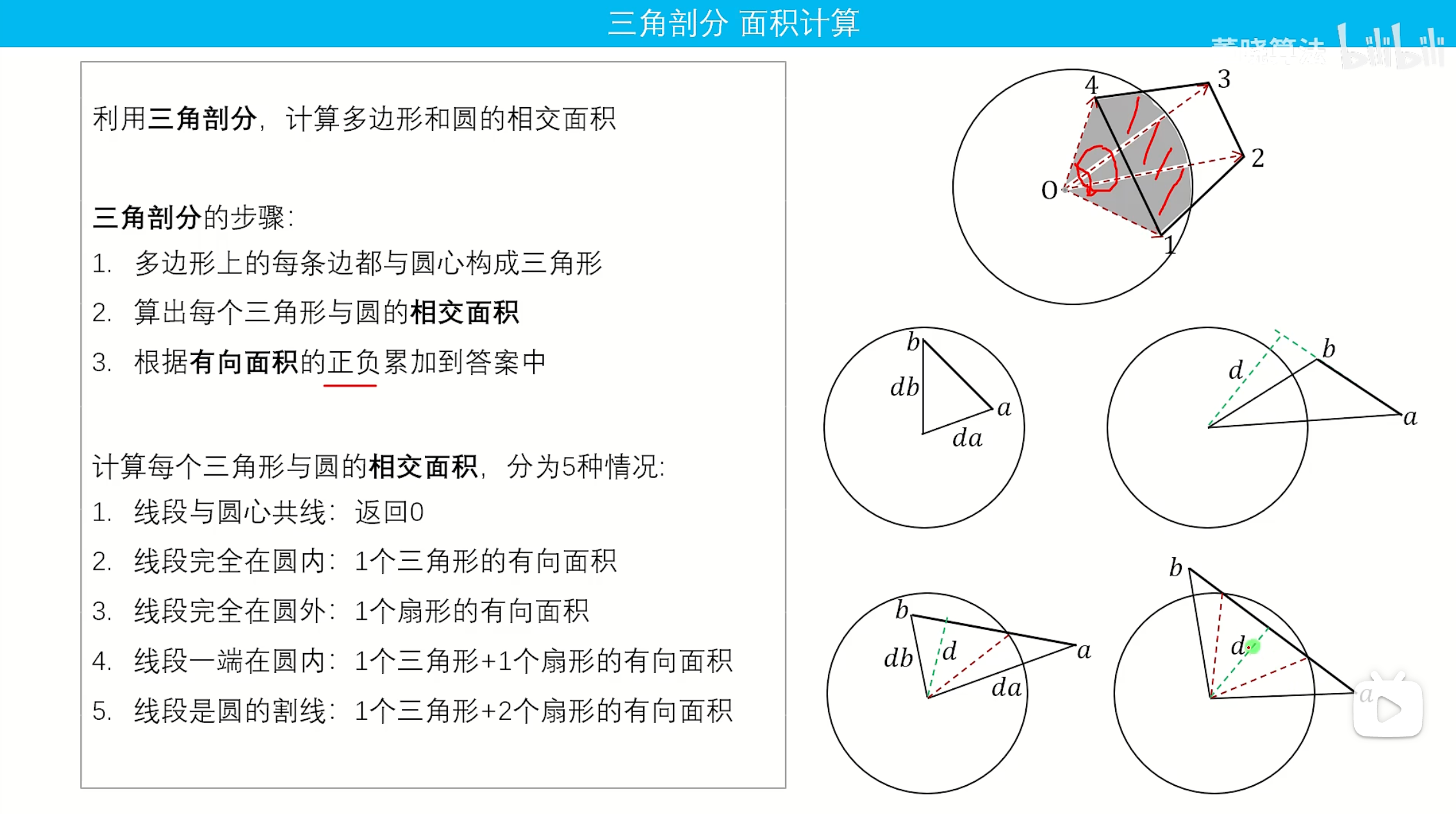
三角剖分